\( \ddot{\theta}=-\dfrac{g}{L}\sin\theta \)
The universe is written in the language of mathematics (Galileo)

|
Solve pendulum
equation
\( \ddot{\theta}=-\dfrac{g}{L}\sin\theta \) The universe is written in the language of mathematics (Galileo) |
| Class schedule by chapters/sections (tentative) | |||
|---|---|---|---|
| Mechanical vibrations:Spring-mass and Pendulum motion (Chapter 1) | 1.1 - 1.3, 1.4* | ||
| Heat conduction: diffusion, convection and advection (Chapter 2) | 2.1 - 2.3* | ||
| Vibration of an elastic string/membrane (Chapter 3*) | 3.1-3.5* | ||
| Exam 1 | Chap.1, 2 and 3* | ||
| Separation of variables: Laplace equation and eigenvalue problem (Chapter 4) | 4.1-4.2,4.3*-4.4* | ||
| Fourier series and integrals (Chapter 5) | 5.1-5.2, 5.3* | ||
| Population dynamics (Chapter 6) | 6.1-6.2, 6.3** | ||
| **Traffic flow (Euler system) (Chapter 7**) | 7.1*, 7.2-7.3* | ||
| Exam 2 | Chap.4, 5 and part of Chap.6* & 7** | ||
| **Numerical Simulations | 8.1**-8.2** | ||
| Final Exam May 6, Thursday 3-5 pm | |||
| Review | Exam | Date |
|---|---|---|
| Review Exam I | Exam I | |
| Review Exam II | Exam II | |
| Review Exam III | Exam III | |
| Review Final | Final Exam |
| Reading-Tutorial | Reading/Tutorial | Chapter/Section |
|---|---|---|
| Reading I | Tutorial I | |
| Reading II | Tutorial II | |
| Reading III | Tutorial III | |
| Reading IV | Tutorial IV |
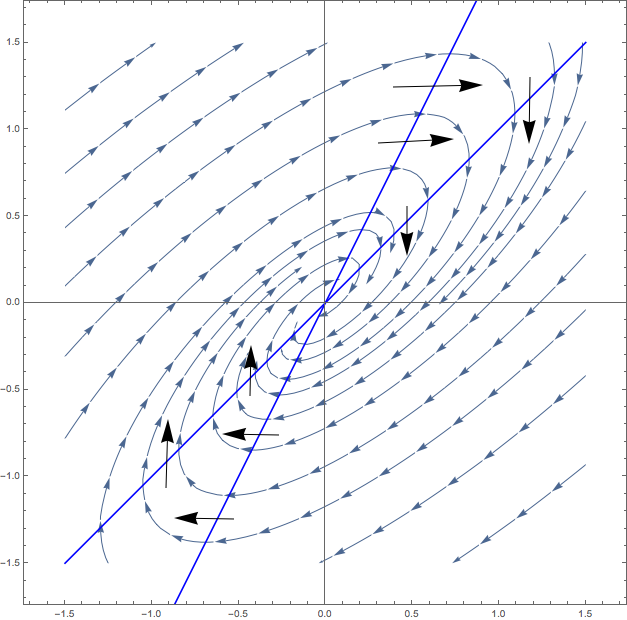
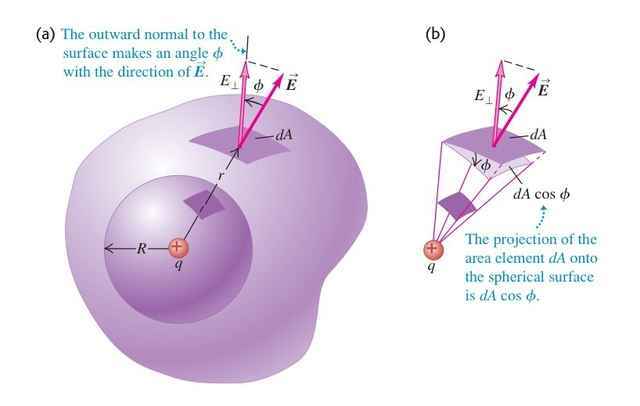
| Green's theorem (2d):
\begin{align*} &\int_{\partial R}\mathbf{F}\cdot \mathbf{n} ds=\int_R \mathrm{div}\mathbf{F} dA \end{align*} The divergence theorem in 3d (Gauss' theorem): \begin{align*} \int_{\partial\Omega}\mathbf{F}\cdot \mathbf{n} d\omega=\int_{\Omega} \mathrm{div} {\bf F} dV\end{align*} |
 Classical harmonic oscillator Classical harmonic oscillator
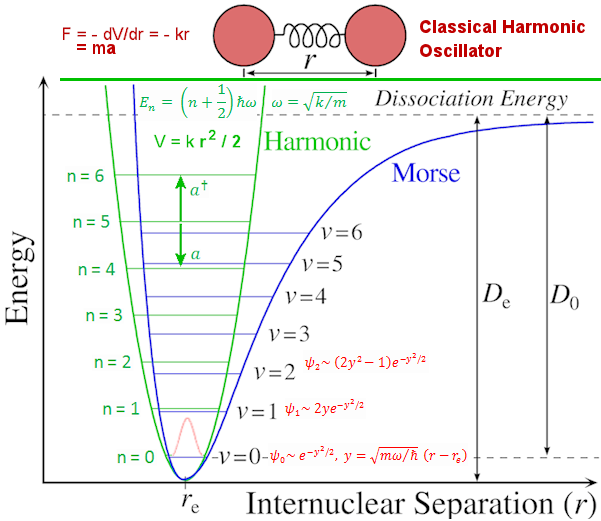
|  Quantum oscillator levels Quantum oscillator levels
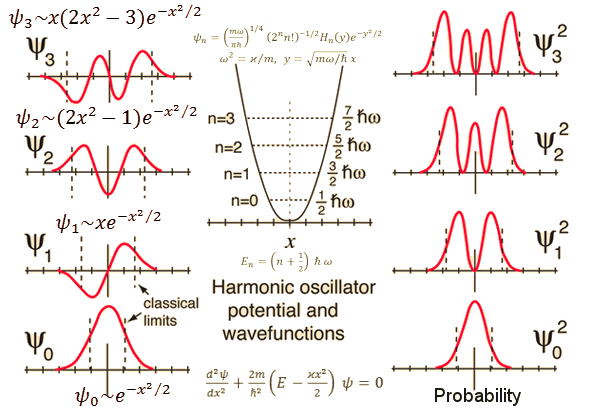
|